The Ultimate TI-84 Calculator Program for SAT® Math

I introduce and cover the features of The College Panda Calculator Program in the TI-84 SAT^® Math video series. If you haven't seen it already, go check that out first. I go through the following:

$TI 84 Plus Graphing Calculator$

Calculator Shortcuts and Basics
Evaluating Expressions
Graphing
How to Download Calculator Programs
The College Panda Program (introduction)
The Rest of The College Panda Program

Watch the video series first.

The College Panda's Calculator Program

This program was specifically designed for the SAT—all its features were created with past questions in mind. We didn't include anything that doesn't help you on the exam. It's the closest thing to a cheat code for the SAT.

When you run it, the first thing you'll see is the main menu.

Main Menu

$Fundamentals Menu$

The program consists of 5 main components:

Fundamentals: basic math operations you may encounter on the exam
Lines: slope, $x$-intercept, $y$-intercept, standard form
Systems of Two Equations: solves systems of 2 equations in standard form
Quadratics: quadratic formula (roots), vertex form, vertex, $y$-intercept, discriminant
Circles: arc length, area of a sector, equation of a circle, center, radius, whether a point is inside a circle

Fundamentals

$Fundamentals Menu$

Features:

Simplify any radical
Pythagorean theorem
Percent change
Distance between 2 points
Midpoint
Convert between radians/degrees

Lines

$Lines Menu$

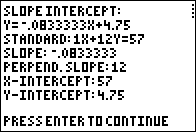$Lines Output$

Input any of the following:

2 points
A point and a slope
Standard form of a line
Slope-intercept form of a line

to get all of the following:

The slope-intercept form of the line
The standard form of the line
The slope of the line
The slope of a perpendicular line
The $x$-intercept of the line
The $y$-intercept of the line

Systems of Two Equations

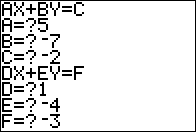
$Systems of Equations Input$ $Systems of Equations Output$

Solves any system of two equations expressed in $ax + by = c$ and $dx + ey = f$ form
Indicates whether the system has infinite solutions
Indicates whether the system has no solutions

Quadratics

$Quadratics Options$

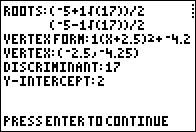$Quadratics Output$

Input any of the following:

Standard form of a quadratic: $ax^2 + bx + c$
Vertex form of a quadratic: $a(x + b)^2 + c$
Factored form of a quadratic: $a(x + b)(x + c)$

to get all of the following:

The roots/solutions (does the quadratic formula for you)
Vertex form (completes the square for you)
The vertex
The discriminant
The $y$-intercept

Circles

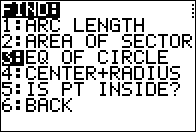
$Circles Menu$ $Circles Equations Options$

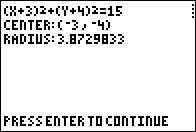
$Circles Equations Input$ $Circles Equations Output$

Arc length
Area of a sector
Equation of a circle from a raw equation ($x^2 + y^2 + ax + by + c = 0$)
Equation of a circle from its center and radius
Equation of a circle from the endpoints of a diameter
The center and radius of a circle from its equation
Checks whether a point is inside or outside a circle in the coordinate plane

Companion Calculator Workbook

It's not enough to just have a program on your calculator. You need to know how and when to use it. This workbook, which contains exercises based on past SAT questions, will teach you how to use the program and for which question types.

$SAT Calculator Workbook$

Chapters:

The Basics (based on part 1 of the video series)
Evaluating Expressions (based on part 2 of the video series)
Graphing (based on part 3 of the video series)
Systems of Equations (based on part 4 of the video series)
Lines (based on part 5 of the video series)
Fundamental Programs (based on part 6 of the video series)
Quadratics (based on part 6 of the video series)
Circles (based on part 6 of the video series)
Answer Explanations

How to Get the Program and Workbook

The Calculator Program and the Workbook are special bonuses for people who have supported my work. Check out the bonuses page for full details on how to get them.